当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
2776:Cheat at Math
题目描述
In Nanjing, a great many students cheat at Mathematics Olympiad contest. Some of them stole the problems. What is worse, some others, like K.W., could even modify the answer sheet to enlarge his scoring. Even though, he is waiting for your help to solve such a complex puzzle, for he cannot solve it himself.
There are four kinds of arcs on the plane: (4 denotes a full circle, 3 denotes ¾ a circle, 2 denotes half a circle, 1 denotes ¼ a circle)
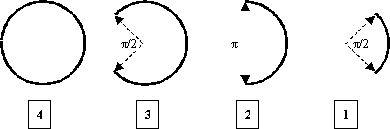
All of the arcs are facing leftwards (as the picture above, one should not rotate them). You are given N <= 1000 arcs on the plane, how many regions are formed out by them (several close regions including the outmost open region)? See the following example:
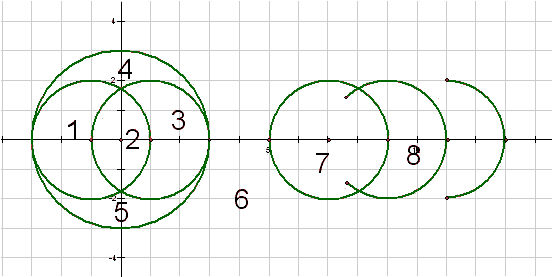
Eight regions are formed out with six arcs.
There are four kinds of arcs on the plane: (4 denotes a full circle, 3 denotes ¾ a circle, 2 denotes half a circle, 1 denotes ¼ a circle)

All of the arcs are facing leftwards (as the picture above, one should not rotate them). You are given N <= 1000 arcs on the plane, how many regions are formed out by them (several close regions including the outmost open region)? See the following example:
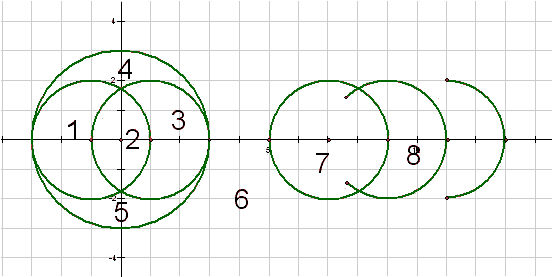
Eight regions are formed out with six arcs.
输入解释
One non-negative integer N <= 1000 occupies the first line. On each of the 2nd ~ (N + 1)-th line, there will be four integers Xi, Yi, Ri, Ti. An arc of type Ti (1 <= Ti <= 4) with radius of Ri is situated on coordinate (Xi, Yi). We have –5000 <= Xi, Yi <= 5000, 1 <= Ri <= 5000.
输出解释
A single line denoting the number of regions formed by those arcs.
输入样例
6 -1 0 2 4 1 0 2 4 0 0 3 4 7 0 2 4 9 0 2 3 11 0 2 2
输出样例
8
提示
Pay attention to the limit of memory.
来自北京大学POJ的附加信息
| Case time limit(单组数据时间限制) | 1000MS |
最后修改于 2020-10-29T06:43:09+00:00 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 10000 | 1024 |
登陆或注册以提交代码