当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
1445:Random number
题目描述
A Black Box algorithm supposes that natural number sequence u(1), u(2), ..., u(N) is sorted in non-descending order, N <= M and for each p (1 <= p <= N) an inequality p <= u(p) <= M is valid.
Making tests for this algorithm we have met with the following problem. For setting a random sequence {u(i)} a usual random data generator did not fit. As the sequence itself had been imposed certain restrictions, the method of choosing the next random element (in the interval defined by restrictions) did not give the random sequence as a whole.
We have come to a conclusion that the problem can be solved in the following way. If we arrange all possible sequences in certain order (for example, in lexicographical order) and assign each sequence its number, after choice of the random number it is possible to take the correspondent sequence for the random one. At the first glance it seems enough to make up a program generating all these sequences in such order. Alas! Even having not great values of M and N it would have taken any powerful modern computer centuries to enumerate all such sequences. It turned out it was possible to avoid generating all sequences if we managed to create required sequence according to its number immediately. But even this statement does not cover all. As the amount of sequences is quite large, the number can be a long one, composed of hundreds decimal digits, though our random data generator could give only normal numbers. We decided to produce a long random number from a real random number distributed in [0,1]. Namely, present the number in binary notation: 0.b(1)b(2)..., where all b(i) = 0 or 1. Let us set a regulation to associate such real number to an integer from [A,B] segment:
Formula 1:
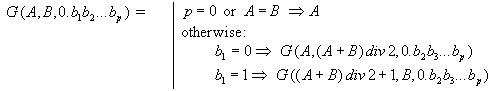
Here we suppose, that A <= B, p >= 0, and ``div 2" is an integer division by 2.
Let M, N (1 <= N <= M <= 200) and a binary real number 0.b(1)b(2)...b(p) (1 <= p <= 400) be given. Write a program to find out the corresponding u(1), u(2), ..., u(N) sequence, i.e. to find a sequence with G(1,T,0.b(1)b(2)...b(p)) number in lexicographical order of all possible {u(i)} for the given M and N (T is the quantity of such sequences). Numeration begins with 1. Keep in mind that in lexicographical order {l(i)} proceeds {h(i)} if after omitting equal beginnings, the first number of {l(i)} tail is smaller than the first number or {h(i)} tail. Following example illustrates the list of all possible sequences for M = 4 and N = 3 in lexicographical order.
A note (it does not concern the solution of this task):
The choice of random binary vector 0.b(1)b(2)...b(p) does not give an absolute uniform random data generator if we use the Formula. However, taking into account the fact that [A,B] interval is big we shall obtain a distribution applicable in most cases.
Example
(here T=14)
Making tests for this algorithm we have met with the following problem. For setting a random sequence {u(i)} a usual random data generator did not fit. As the sequence itself had been imposed certain restrictions, the method of choosing the next random element (in the interval defined by restrictions) did not give the random sequence as a whole.
We have come to a conclusion that the problem can be solved in the following way. If we arrange all possible sequences in certain order (for example, in lexicographical order) and assign each sequence its number, after choice of the random number it is possible to take the correspondent sequence for the random one. At the first glance it seems enough to make up a program generating all these sequences in such order. Alas! Even having not great values of M and N it would have taken any powerful modern computer centuries to enumerate all such sequences. It turned out it was possible to avoid generating all sequences if we managed to create required sequence according to its number immediately. But even this statement does not cover all. As the amount of sequences is quite large, the number can be a long one, composed of hundreds decimal digits, though our random data generator could give only normal numbers. We decided to produce a long random number from a real random number distributed in [0,1]. Namely, present the number in binary notation: 0.b(1)b(2)..., where all b(i) = 0 or 1. Let us set a regulation to associate such real number to an integer from [A,B] segment:
Formula 1:
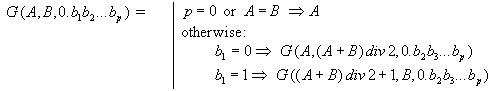
Here we suppose, that A <= B, p >= 0, and ``div 2" is an integer division by 2.
Let M, N (1 <= N <= M <= 200) and a binary real number 0.b(1)b(2)...b(p) (1 <= p <= 400) be given. Write a program to find out the corresponding u(1), u(2), ..., u(N) sequence, i.e. to find a sequence with G(1,T,0.b(1)b(2)...b(p)) number in lexicographical order of all possible {u(i)} for the given M and N (T is the quantity of such sequences). Numeration begins with 1. Keep in mind that in lexicographical order {l(i)} proceeds {h(i)} if after omitting equal beginnings, the first number of {l(i)} tail is smaller than the first number or {h(i)} tail. Following example illustrates the list of all possible sequences for M = 4 and N = 3 in lexicographical order.
A note (it does not concern the solution of this task):
The choice of random binary vector 0.b(1)b(2)...b(p) does not give an absolute uniform random data generator if we use the Formula. However, taking into account the fact that [A,B] interval is big we shall obtain a distribution applicable in most cases.
Example
1, 2, 3
1, 2, 4
1, 3, 3
1, 3, 4
1, 4, 4
2, 2, 3
2, 2, 4
2, 3, 3
2, 3, 4
2, 4, 4
3, 3, 3
3, 3, 4
3, 4, 4
4, 4, 4
(here T=14)
输入解释
The first line of input contains M and N. The second line contains binary real number 0.b(1)b(2)...b(p) (without leading, trailing and other spaces).
输出解释
Write into the output the corresponding sequence u(1), u(2), ..., u(N). The sequence numbers should be separated with one space.
输入样例
4 3 0.01101101011110010001101010001011010
输出样例
2 2 4
提示
A note (it does not concern the solution of this task):
The choice of random binary vector 0.b(1)b(2)...b(p) does not give an absolute uniform random data generator if we use Formula 1. However, taking into account the fact that [A,B] interval is big we shall obtain a distribution applicable in most cases.
The choice of random binary vector 0.b(1)b(2)...b(p) does not give an absolute uniform random data generator if we use Formula 1. However, taking into account the fact that [A,B] interval is big we shall obtain a distribution applicable in most cases.
最后修改于 2020-10-29T06:04:18+00:00 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 3000 | 10000 |
登陆或注册以提交代码