当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
6450:Insertion Sort
题目描述
Insertion sort is a simple sorting algorithm that builds the final sorted array one item at an iteration.
More precisely, insertion sort iterates, consuming one input element each repetition, and growing a sorted output list.
At each iteration, insertion sort removes one element from the input data, finds the location it belongs within the sorted list, and inserts it there.
It repeats until no input elements remain.
This type of sorting is typically done in-place, by iterating up the array, growing the sorted array behind it.
At each array-position, it checks the value there against the largest value in the sorted array (which happens to be next to it, in the previous array-position checked).
If larger, it leaves the element in place and moves to the next.
If smaller, it finds the correct position within the sorted array, shifts all the larger values up to make a space, and inserts into that correct position.
The resulting array after $k$ iterations has the property where the first $k$ entries are sorted.
In each iteration the first remaining entry of the input is removed, and inserted into the result at the correct position, thus extending the result.
Knuth is an ACM-ICPC master and provides a modified pseudocode implementation about the insertion sort for you.
His modified algorithm for an array of sortable items $A$ ($1$-based array) can be expressed as:
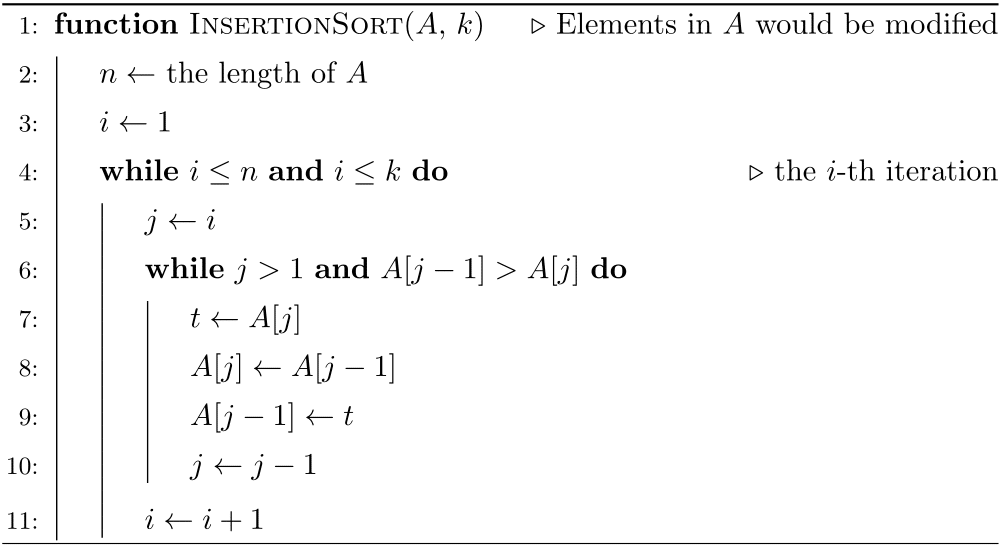
Given the parameter $k$, you are asked to count the number of distinct permutations of $1$ to $n$ meeting the condition that, after his modified insertion sort, each permutation would become an almost sorted permutation.
Here he notes that a permutation of $1$ to $n$ is almost sorted if the length of its longest increasing subsequence is at least $(n - 1)$.
More precisely, insertion sort iterates, consuming one input element each repetition, and growing a sorted output list.
At each iteration, insertion sort removes one element from the input data, finds the location it belongs within the sorted list, and inserts it there.
It repeats until no input elements remain.
This type of sorting is typically done in-place, by iterating up the array, growing the sorted array behind it.
At each array-position, it checks the value there against the largest value in the sorted array (which happens to be next to it, in the previous array-position checked).
If larger, it leaves the element in place and moves to the next.
If smaller, it finds the correct position within the sorted array, shifts all the larger values up to make a space, and inserts into that correct position.
The resulting array after $k$ iterations has the property where the first $k$ entries are sorted.
In each iteration the first remaining entry of the input is removed, and inserted into the result at the correct position, thus extending the result.
Knuth is an ACM-ICPC master and provides a modified pseudocode implementation about the insertion sort for you.
His modified algorithm for an array of sortable items $A$ ($1$-based array) can be expressed as:

Given the parameter $k$, you are asked to count the number of distinct permutations of $1$ to $n$ meeting the condition that, after his modified insertion sort, each permutation would become an almost sorted permutation.
Here he notes that a permutation of $1$ to $n$ is almost sorted if the length of its longest increasing subsequence is at least $(n - 1)$.
输入解释
The input contains several test cases, and the first line contains a positive integer $T$ indicating the number of test cases which is up to $5000$.
For each test case, the only line contains three integers $n, k$ and $q$ indicating the length of the permutations, the parameter in his implementation and a prime number required for the output respectively, where $1 \leq n, k \leq 50$ and $10^8 \leq q \leq 10^9$.
For each test case, the only line contains three integers $n, k$ and $q$ indicating the length of the permutations, the parameter in his implementation and a prime number required for the output respectively, where $1 \leq n, k \leq 50$ and $10^8 \leq q \leq 10^9$.
输出解释
For each test case, output a line containing "Case #x: y" (without quotes), where $x$ is the test case number starting from $1$, and $y$ is the remainder of the number of permutations which meet the requirement divided by $q$.
输入样例
4 4 1 998244353 4 2 998244353 4 3 998244353 4 4 998244353
输出样例
Case #1: 10 Case #2: 14 Case #3: 24 Case #4: 24
提示
In the first sample case, we can discover 10 permutations which meet the condition, and they are listed as follows: [1, 2, 3, 4] [1, 2, 4, 3] [1, 3, 2, 4] [1, 3, 4, 2] [1, 4, 2, 3] [2, 1, 3, 4] [2, 3, 1, 4] [2, 3, 4, 1] [3, 1, 2, 4] [4, 1, 2, 3]
来自杭电HDUOJ的附加信息
| Recommend | chendu |
最后修改于 2020-10-25 23:31:27 UTC 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 2000/1000MS(Java/Others) | 262144/262144K(Java/Others) |
登陆或注册以提交代码