当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
4227:Hexagram
题目描述
A Hexagram is a 6-pointed star, sometimes called the Star of David. Given these numbers:
3 17 15 18 11 22 12 23 21 7 9 13
There are four unique ways of assigning the numbers to vertices of the hexagram such that all of the sets of four numbers along the lines have the same sum (57 in this case). All other ways may be obtained from these by rotation and/or reflection.
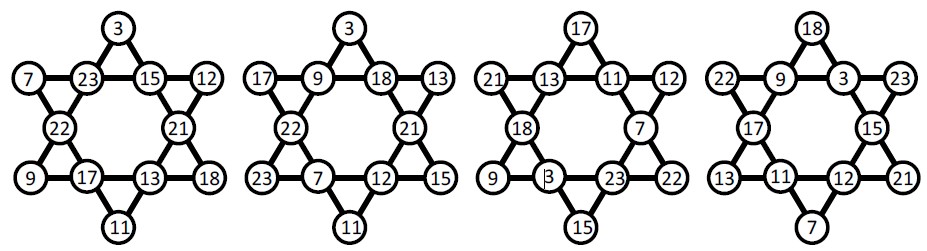
Given 12 distinct numbers, in how many ways, disregarding rotations and reflections, can you assign the numbers to the vertices such that the sum of the numbers along each of 6 straight lines passing through 4 vertices is the same?
There are four unique ways of assigning the numbers to vertices of the hexagram such that all of the sets of four numbers along the lines have the same sum (57 in this case). All other ways may be obtained from these by rotation and/or reflection.

Given 12 distinct numbers, in how many ways, disregarding rotations and reflections, can you assign the numbers to the vertices such that the sum of the numbers along each of 6 straight lines passing through 4 vertices is the same?
输入解释
There will be several test cases in the input. Each test case will consist of twelve unique positive integers on a single line, with single spaces separating them. All of the numbers will be less than 1,000,000. The input will end with a line with twelve 0s.
输出解释
For each test case, output the number of ways the numbers can be assigned to vertices such that the sum along each line of the hexagram is the same. Put each answer on its own line. Output no extra spaces, and do not separate answers with blank lines.
输入样例
3 17 15 18 11 22 12 23 21 7 9 13 1 2 3 4 5 6 7 8 9 10 11 13 0 0 0 0 0 0 0 0 0 0 0 0
输出样例
4 0
来自杭电HDUOJ的附加信息
| Recommend | lcy |
最后修改于 2020-10-25T23:11:30+00:00 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 2000/1000MS(Java/Others) | 32768/32768K(Java/Others) |
登陆或注册以提交代码