当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
3694:Fermat Point in Quadrangle
题目描述
In geometry the Fermat point of a triangle, also called Torricelli point, is a point such that the total distance from the three vertices of the triangle to the point is the minimum. It is so named because this problem is first raised by Fermat in a private letter. In the following picture, P0 is the Fermat point. You may have already known the property that:
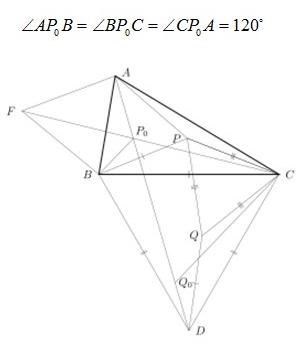
Alice and Bob are learning geometry. Recently they are studying about the Fermat Point.
Alice: I wonder whether there is a similar point for quadrangle.
Bob: I think there must exist one.
Alice: Then how to know where it is? How to prove?
Bob: I don’t know. Wait… the point may hold the similar property as the case in triangle.
Alice: It sounds reasonable. Why not use our computer to solve the problem? Find the Fermat point, and then verify your assumption.
Bob: A good idea.
So they ask you, the best programmer, to solve it. Find the Fermat point for a quadrangle, i.e. find a point such that the total distance from the four vertices of the quadrangle to that point is the minimum.

Alice and Bob are learning geometry. Recently they are studying about the Fermat Point.
Alice: I wonder whether there is a similar point for quadrangle.
Bob: I think there must exist one.
Alice: Then how to know where it is? How to prove?
Bob: I don’t know. Wait… the point may hold the similar property as the case in triangle.
Alice: It sounds reasonable. Why not use our computer to solve the problem? Find the Fermat point, and then verify your assumption.
Bob: A good idea.
So they ask you, the best programmer, to solve it. Find the Fermat point for a quadrangle, i.e. find a point such that the total distance from the four vertices of the quadrangle to that point is the minimum.
输入解释
The input contains no more than 1000 test cases.
Each test case is a single line which contains eight float numbers, and it is formatted as below:
x1 y1 x2 y2 x3 y3 x4 y4
xi, yi are the x- and y-coordinates of the ith vertices of a quadrangle. They are float numbers and satisfy 0 ≤ xi ≤ 1000 and 0 ≤ yi ≤ 1000 (i = 1, …, 4).
The input is ended by eight -1.
Each test case is a single line which contains eight float numbers, and it is formatted as below:
x1 y1 x2 y2 x3 y3 x4 y4
xi, yi are the x- and y-coordinates of the ith vertices of a quadrangle. They are float numbers and satisfy 0 ≤ xi ≤ 1000 and 0 ≤ yi ≤ 1000 (i = 1, …, 4).
The input is ended by eight -1.
输出解释
For each test case, find the Fermat point, and output the total distance from the four vertices to that point. The result should be rounded to four digits after the decimal point.
输入样例
0 0 1 1 1 0 0 1 1 1 1 1 1 1 1 1 -1 -1 -1 -1 -1 -1 -1 -1
输出样例
2.8284 0.0000
来自杭电HDUOJ的附加信息
| Recommend | axubiao |
最后修改于 2020-10-25T23:06:08+00:00 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 2000/1000MS(Java/Others) | 65536/32768K(Java/Others) |
登陆或注册以提交代码