当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
3441:Rotation
题目描述
As you know, AekdyCoin loves games.One day,he got a small square board such as the one in Figure 1,the length of side is A (Here A is an integer!).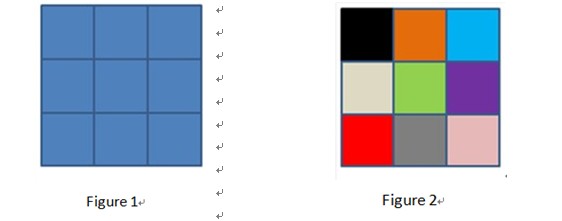
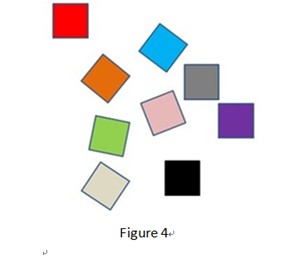
If one board could be rotated to another board (both painted), we consider them as the same, just as you could see in the figure 3。
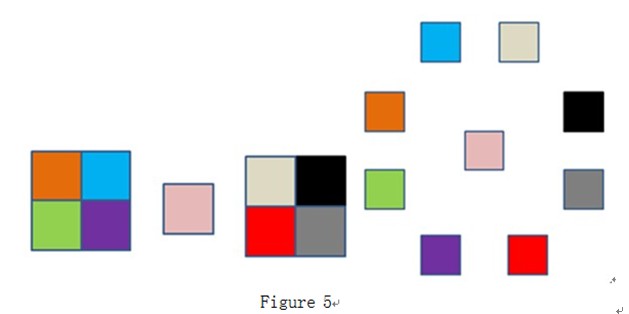
B * B * K + 1 = A * A, (K >= 0)
in the figure 5, there are two possible ways, B = 1 or B = 2
Then AekdyCoin connect the left one 1 * 1 square to other B * B square(s), as in the figure 6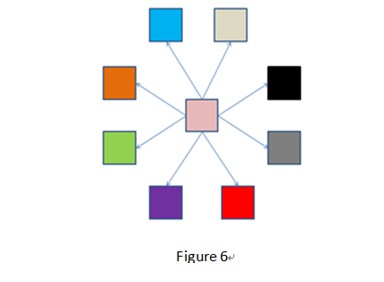
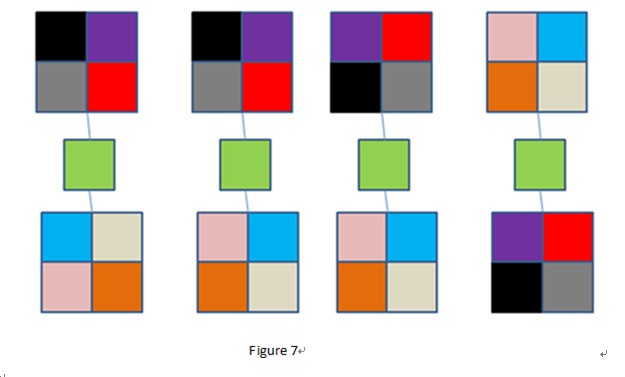

If one board could be rotated to another board (both painted), we consider them as the same, just as you could see in the figure 3。



B * B * K + 1 = A * A, (K >= 0)
in the figure 5, there are two possible ways, B = 1 or B = 2
Then AekdyCoin connect the left one 1 * 1 square to other B * B square(s), as in the figure 6


输入解释
The first line is an integer T indicates the number of the cases. ( T <= 1000)
Then T lines
A C
Indicate the value of A and the kind of colors AekdyCoin has. (1<=A,C<=10^9)
Then T lines
A C
Indicate the value of A and the kind of colors AekdyCoin has. (1<=A,C<=10^9)
输出解释
Output a single integer indicates the remainder of the answer after divided by 1000000007
输入样例
2 2 7 3 2
输出样例
Case 1: 833 Case 2: 114
来自杭电HDUOJ的附加信息
| Author | AekdyCoin |
| Recommend |
最后修改于 2020-10-25T23:03:35+00:00 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 2000/1000MS(Java/Others) | 32768/32768K(Java/Others) |
登陆或注册以提交代码