当前你的浏览器版本过低,网站已在兼容模式下运行,兼容模式仅提供最小功能支持,网站样式可能显示不正常。
请尽快升级浏览器以体验网站在线编辑、在线运行等功能。
3230:Assembling Services
Special Judge 特殊评判题目描述
In this problem, you need to simulate the execution of n service programs P1, P2, ..., Pn. Each program is described with sequence of integers: T I in1 in2 ... inI O out1 out2 ... outO, that means it takes T unit time to execute, needs I input variables (i.e. in1 in2 ... inI), and sets O output variables (i.e. out1 out2 ... outO) when it finishes running. A program can be started if and only if all these T input variables are ready (initially available, or set by some other programs).
Imagine you have a super-computer which can execute as many programs in parallel as you like, and every variable can be read and written simultaneously by multiple programs. Your task is to calculate a particular "target" variable, as soon as possible.
Assume there are 4 programs, shown in the table below:
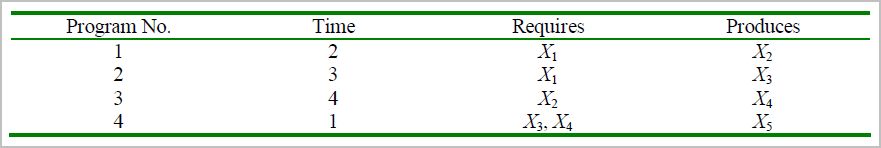
The quickest time to get X5 is 7, if only X1 is available at startup.
You also need to construct an expression that shows how to execute the programs to achieve the minimal time. The grammar of the expression is recursive:
Imagine you have a super-computer which can execute as many programs in parallel as you like, and every variable can be read and written simultaneously by multiple programs. Your task is to calculate a particular "target" variable, as soon as possible.
Assume there are 4 programs, shown in the table below:

The quickest time to get X5 is 7, if only X1 is available at startup.
You also need to construct an expression that shows how to execute the programs to achieve the minimal time. The grammar of the expression is recursive:
- Single Program: Px, where 1 <= x <= n. (i.e. P2, P499, etc). Meaning: execute the program immediately. Then end of this program marks the end of this expression.
- Execute in serial: (S1S2...Sk), where every Si is an expression. Note that the outermost pair of parentheses is mandatory. Meaning: execute expression S1, then S2 immediately after S1 ends, then S3 immediately after S2 ends, ..., and finally Sk immediately after Sk-1 ends. Then end of expression Sk marks the end of the whole expression.
- Execute in parallel: (S1|S2|...|Sk), where every Si is an expression. Note that the outermost pair of parentheses is mandatory. Meaning: execute expressions S1, S2, ..., and Sk simultaneously. The end of last finished expression marks the end of the whole expression.
输入解释
There will be at most 100 test cases. Each case begins with three integers n, m, o(1 <= n,m <= 500, 1 <= o <= m). The number of programs is n, the number of variables is m, and the target variable is Xo. Variables are numbered 1 to m, programs are numbered 1 to n. The next line contains a 01 string of m characters. The i-th character is 1 if and only if the i-th variable is initially available. The target variable is guaranteed to be unavailable at startup. The following n lines describe the programs. Each line begins with an integer T(1 <= T <= 100), the execution time, and an integer I followed by I integers in1, in2, ..., inI, as stated above, then an integer O followed by O integers out1, out2, ..., outO. 1 <= ini,outi <= m, 1 <= I,O <= 10. The last test case is followed by n=m=o=0, which should not be processed.
输出解释
For each test case, print the case number and the total time needed to get the target variable. If it's not possible to get the target variable, print -1 in stead.
If it's possible to get the target variable, print the expression after that, in the same line. Be sure to print a valid expression having at most 10,000 characters, with each program printed at most once. There should be no whitespace characters within the expression.
To make this problem a little bit easier, it's allowed that some programs finish after the optimal time, as long as the target variable is available at the optimal time. You’re also allowed to print redundant parentheses (pay attention to the expression length, though). If such an expression does not exist, print "Can't do in serial-parallel.", without quotes.
Print a blank line after the output of each test case.
If it's possible to get the target variable, print the expression after that, in the same line. Be sure to print a valid expression having at most 10,000 characters, with each program printed at most once. There should be no whitespace characters within the expression.
To make this problem a little bit easier, it's allowed that some programs finish after the optimal time, as long as the target variable is available at the optimal time. You’re also allowed to print redundant parentheses (pay attention to the expression length, though). If such an expression does not exist, print "Can't do in serial-parallel.", without quotes.
Print a blank line after the output of each test case.
输入样例
4 5 5 10000 2 1 1 1 2 3 1 1 1 3 4 1 2 1 4 1 2 3 4 1 5 1 2 1 01 31 1 2 1 1 3 5 5 10100 3 1 1 1 2 1 1 3 1 4 3 2 4 2 1 5 1 3 3 100 1 1 1 1 2 0 0 0
输出样例
Case 1: 7 (((P1P3)|P2)P4) Case 2: 31 P1 Case 3: 6 ((P1P3)|P2) Case 4: -1
提示
Explanation After a variable is set, it'll keep available forever. That's why P3 can be executed, in the third example. Also note that there are some other correct expressions for the first sample, e.g.((P1P3P4)|P2). You can even print (((P1P3)P4)|P2) or ((P1(P3P4))|P2). Any one of them is acceptable in this problem.
来自杭电HDUOJ的附加信息
| Recommend | chenrui |
最后修改于 2020-10-25T23:01:27+00:00 由爬虫自动更新
共提交 0 次
通过率 --%
| 时间上限 | 内存上限 |
| 2000/1000MS(Java/Others) | 32768/32768K(Java/Others) |
登陆或注册以提交代码